Sensitivity (updated Sep 2023)

This page provides the information on instrumental sensitivities necessary to plan MODS spectroscopic and imaging observations. The zeropoints were updated in September 2023. The model LBT atmospheric extinction curve was updated in November 2024.

There have been gradual changes in the sensitivities of both instruments (or rather the instrument+telescope, which is what was measured) over time, most dramatically for MODS1, on the LBT-SX side, whose sensitivity has declined by ~1 magnitude since the instrument was commissioned in 2011.

One of the contributors to the changing MODS1+LBT-SX sensitivity has been the degradation of the coating on the SX adaptive secondary (ASM). On 25 May 2023, the SX ASM was removed and replaced by the rigid secondary, and in September 2023, we went back on-sky after the summer shutdown with the freshly recoated ASM on the SX side. This page contains the MODS grating and imaging zeropoints measured on 26 May 2023 UT and most recently on 06 Sep 2023 UT. Between these measurements, besides the changes to the SX M2, the DX primary was recoated and the SX primary was washed.

Comparison of the rigid M2 and ASM may be found on the page updated in June 2023, and a comparison of the September zeropoints with those from June and earlier will be added to this page soon. For now, it is noted that from February 2023 to September 2023, the direct mode throughput increased by 87%, 70% and 45% at 4500, 5000 and 6450 angstroms, which is roughly consistent with the improvement in the measured reflectivity of the adaptive secondary.

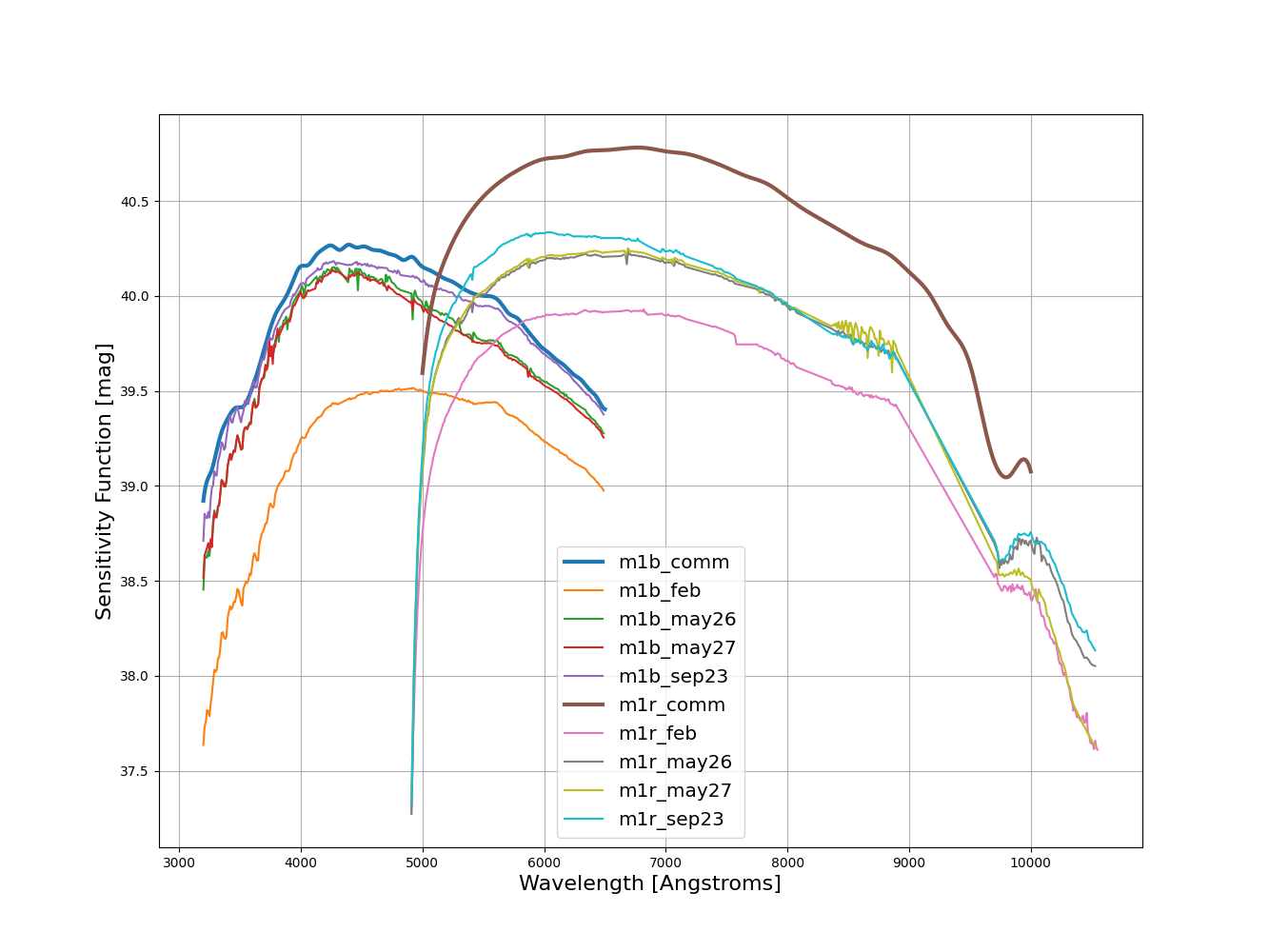
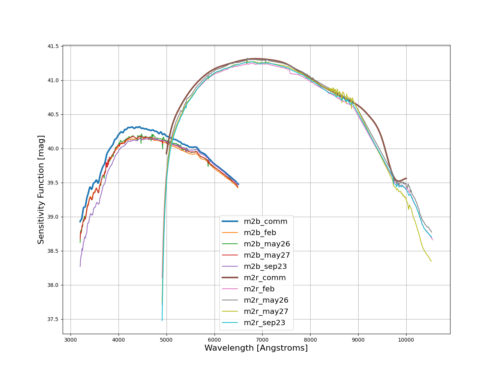
Figure 1:  The evolution of the sensitivities for the direct modes of MODS1+LBT-SX (top) and MODS2+LBT-DX (bottom), from their respective commissionings in 2011 & 2015, a night in February 2023 with the SX and DX ASMs, 26&27 May 2023 after the SX ASM was swapped out for the rigid secondary, and finally from September 2023, after the freshly recoated ASM was reinstalled on the SX side.

With the secondary mirror swap, the relative photon flux ratio of MODS1 with respect to MODS2 is now 0.9-1 at the blue end, but at the red end, it is about 0.5-0.6.  Assuming the same relative flux ratios for signal and sky, and that the dominant source of noise is the sky background, then to achieve the same SNR with MODS1 as with MODS2 in the red would require an exposure that is almost twice as long.

The grating and imaging zeropoints, along with the grating signal-to-noise tracks, have been updated using the 06 Sep 2023 data.

Spectroscopic Mode

The number of counts per angstrom that may be expected from a source with flux, F_{λ ,} at a given wavelength, airmass and exposure time, can be estimated using the following formula and the zeropoint at that wavelength, log S_{λ ,0}.

log S_λ = log S_{λ ,0}  + log F_λ  + log texp – 0.4  K_λ  X

where:

log S_λ = log counts in ADU Å^-1 at wavelength λ
log S_{λ ,0} = Spectroscopic zero point in ADU Å^-1at wavelength, see tables below.
F_λ = flux in units of erg/sec/cm²/Å
t_exp = exposure time in seconds
K_λ = estimated extinction coefficient at wavelength λ
X = airmass at the time of the observation

To convert AB magnitudes to F_λ:

log F_λ = -0.9608 – 0.4 AB – 2 log λ

where AB = Spectral AB magnitude and λ is the wavelength in Angstroms.

The spectroscopic zero points at selected wavelengths for the MODS red and blue grating and prism modes are listed in the Tables 1a and 2 below. For a given source flux (F_λ), exposure time and airmass, these allow a 10-20% prediction of counts per angstrom, S_λ, which can be converted to counts per pixel when multiplied by the spectral pixel size in Å. The effect of atmospheric extinction is factored in by selecting a nominal airmass for the observation and applying an appropriate model for the atmospheric extinction (see below).  A caveat — predictions based on these zero points should be considered as for the best-case scenario, as there are various observational factors that can degrade the sensitivity achieved: slit losses, which depend on seeing among other factors; changes in sky brightness with time, telescope pointing or moon phase and distance from target; changes in transparency; and changes in the reflectivities of the telescope or instrument optics, are some examples.

Grating Mode

The efficiencies for the grating spectroscopic modes, based on MODS1+SX and MODS2+DX data from May 26, 2023 UT. These efficiencies are for MODS1 plus the LBT SX primary and secondary mirrors and MODS2 plus the LBT DX primary and secondary mirrors and are exclusive of the atmosphere (i.e. using the diameters of the primaries and their central obscurations, but assuming perfect reflectivities and assume airmass=0). The efficiency of MODS1+SX has decreased by approximately a factor of 2 since the time of commissioning when efficiencies were similar to those of MODS2. The decrease is wavelength-dependent. Also, the red-only efficiencies of both MODS1 and MODS2 have decreased with respect to the dual modes above 6000 or 7000 angstroms, possibly due to some degradation of the Ag coatings on the flat mirrors in the red channel. The upturn in red-only modes above 9700 Angstroms is due to 2nd order light that is not blocked by the GG495 filter which has a cut-on wavelength of 4950 Angstroms.

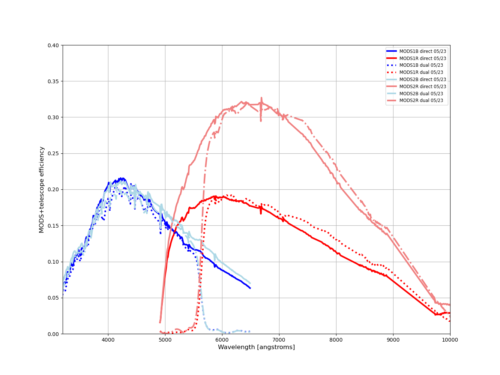
Figure 2: MODS1+SX and MODS2+DX airmass=0 efficiencies for direct (solid) and dual (dotted) grating modes from data taken in May 2023 (to be updated to use data from Sep 2023, after the SX ASM was recoated). The top pair of blue & red curves is for MODS2 and the bottom, for MODS1. All curves assume perfect reflectivity for the primary and secondary mirrors, which is not be realistic, and so the value of the plot is mainly in illustrating the relative instrument+telescope throughputs.

 

The replacement of the SX ASM with the rigid secondary improved the sensitivity of MODS1+LBT-SX, but while the blue end recovered to almost the level at commissioning and is now comparable to MODS2+LBT-DX, the red end saw a smaller improvement.

Zeropoints

The spectroscopic zero points as a function of wavelength for the MODS1 and MODS2 grating modes, log S_{λ ,0,} are given in Table 1a below. These are based on data taken on 20230906 UT, after the freshly recoated adSec was installed on the SX side. For comparison, Table 1b shows the zeropoints from 20230526 UT, after the SX adSec was swapped out for the rigid secondary.

Table1a: MODS1+LBT-SX and MODS2+LBT-DX Grating Mode Spectroscopic Zero Points (6 Sep 2023 UT)

	MODS1 Red Grating		MODS2 Red grating			MODS1 Blue Grating		MODS2 Blue grating
λ(Å)	Direct logSλ,0	Dichroic log Sλ ,0	Direct logSλ,0	Dichroic log Sλ ,0	λ(Å)	Direct logSλ,0	Dichroic log Sλ ,0	Direct logSλ,0	Dichroic log Sλ ,0
5000	15.67	…	15.79	…	3200	15.484	15.436	15.308	15.247
5500	16.072	14.595	16.288	14.989	3500	15.742	15.718	15.655	15.629
6000	16.132	16.126	16.441	16.425	4000	16.026	15.994	15.983	15.938
6500	16.122	16.133	16.491	16.486	4500	16.065	16.046	16.05	16.032
7000	16.094	16.109	16.499	16.503	5000	16.03	16.03	16.038	16.03
7500	16.05	16.095	16.473	16.502	5500	15.978	15.961	15.989	15.954
8000	15.981	16.041	16.403	16.441	5800	15.932	14.647	15.958	14.615
8500	15.913	15.969	16.317	16.347	6000	15.878	…	15.904	…
9000	15.819	15.877	16.203	16.23	6450	15.766	…	15.799	…
9500	15.581	15.634	15.947	15.977
10000	15.208(*)	15.268	15.524(*)	15.554

Table1b: MODS1+LBT-SX and MODS2+LBT-DX Grating Mode Spectroscopic Zero Points (26 May 2023 UT)

	MODS1 Red Grating		MODS2 Red grating			MODS1 Blue Grating		MODS2 Blue grating
λ(Å)	Direct logSλ,0	Dichroic log Sλ ,0	Direct logSλ,0	Dichroic log Sλ ,0	λ(Å)	Direct logSλ,0	Dichroic log Sλ ,0	Direct logSλ,0	Dichroic log Sλ ,0
5000	15.632	…	15.84	…	3200	15.382	15.33	15.448	15.387
5500	16.004	14.504	16.309	14.992	3500	15.688	15.653	15.74	15.712
6000	16.076	16.072	16.455	16.44	4000	16.012	15.972	16.022	15.976
6500	16.083	16.099	16.503	16.501	4500	16.05	16.024	16.069	16.051
7000	16.07	16.089	16.516	16.519	5000	15.985	15.976	16.041	16.035
7500	16.036	16.085	16.493	16.522	5500	15.907	15.882	15.98	15.945
8000	15.977	16.039	16.418	16.456	5800	15.863	14.584	15.94	14.572
8500	15.916	15.975	16.338	16.367	6000	15.82	…	15.888	…
9000	15.82	15.879	16.225	16.251	6450	15.725	…	15.789	…
9500	15.577	15.632	15.967	15.997
10000	15.2(*)	15.264	15.539(*)	15.569

*: In the red direct mode, there is 2nd order contamination above 9700 angstroms and the zeropoint is taken to be the dual grating zeropoint minus the rounded average dual-to-direct offset at the red end of 0.06 dex (MODS1) or 0.03 dex (MODS2).

For comparison, the zeropoints in Table 1b are based on data taken in September and October 2022.

Table1b: MODS1+LBT-SX and MODS2+LBT-DX Grating Mode Spectroscopic Zero Points (Sep-Oct 2022 UT)

	MODS1 Red Grating		MODS2 Red grating			MODS1 Blue Grating		MODS2 Blue grating
λ(Å)	Direct logSλ,0	Dichroic log Sλ ,0	Direct logSλ,0	Dichroic log Sλ ,0	λ(Å)	Direct logSλ,0	Dichroic log Sλ ,0	Direct logSλ,0	Dichroic log Sλ ,0
5000	15.501	…	15.865	…	3200	15.091	15.016	15.485	15.431
5500	15.910	14.420	16.319	15.013	3500	15.388	15.349	15.766	15.746
6000	15.995	15.985	16.465	16.458	4000	15.728	15.681	16.026	15.992
6500	16.001	16.011	16.511	16.516	4500	15.814	15.788	16.070	16.059
7000	15.993	16.005	16.522	16.534	5000	15.819	15.809	16.045	16.044
7500	15.970	16.009	16.505	16.542	5500	15.797	15.763	15.991	15.955
8000	15.902	15.958	16.432	16.477	5800	15.764	14.459	15.956	14.597
8500	15.843	15.897	16.350	16.386	6000	15.719	…	15.902	…
9000	15.753	15.816	16.232	16.268	6450	15.622	…	15.799	…
9500	15.521	15.570	15.965	16.008
10000	15.420	15.208	15.793	15.571

 

SNR tracks

Figures 3.1, 3.2 and 3.3, below, plot the sensitivity limits as a function of time for a given signal-to-noise ratio. These are based on measurements made with MODS1 and MODS2 on May 26, 2023 UT, after the SX ASM was replaced by the rigid secondary. The calculation of these SNR tracks assumes a Gaussian PSF to account for slit losses and to determine the contribution from the sky, where an extraction window of 2.4 * FWHM rows, which would contain 99.5% of the light from an unresolved source, is used.

Figure 3.1 (below): SNR tracks for MODS1 & MODS2 at g and r: For signal-to-noise ratios, SNR= 5, 20 and 100 per 0.5 (blue) or 0.85 angstrom (red) pixel in a spectrum that has been extracted over the number of rows corresponding to the seeing FWHM, tracks of AB magnitude vs time are plotted for both blue (at g_sdss/4770 angstroms) and red (r_sdss/6300 angstroms) channels. These tracks use the MODS1 and MODS2 dual grating zeropoints tabulated here and have been calculated for a 1″ slit, seeing FWHM = 1″, airmass = 1 and dark (new moon, solid lines) and bright (10-d moon, dotted lines) skies. The blue and red shaded regions indicate the range of magnitudes predicted for sky brightnesses from new to 10-d moon. The slit loss calculation assumes a Gaussian PSF, so for a slit width equal to the seeing FWHM, 24 percent of the light is lost. For the sky contribution, the area is the number of pixels covered by the slit width times the FWHM. The dark(bright) sky brightnesses at g_sdss and r_sdss were assumed to be 22.3(20.7) and 21.3(20.6), respectively. These are interpolated from the KPNO sky brightnesses and agree well with published dark sky values for Mt. Graham (Taylor+2004,PASP,116,762 and Pedani 2009, PASP,121,778).

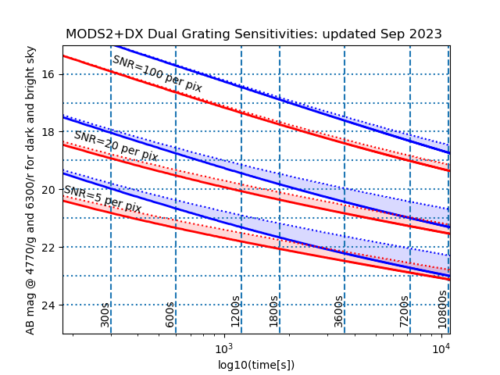
To do a “bye-eye interpolation” from this plot, recall that the SNR scales with the square root of the flux and of the exposure time, although for fainter objects whose flux is comparable to the sky brightness, SNR grows more slowly.

Caveat: The  predicted SNRs should be considered best-case scenarios, as there are various observational factors that can degrade the actual SNR you see in your data: transparency, slit loss, sky brightness which can change with time (in the red, for example) and with telescope pointing (looking at a low elevation target in the direction of a city, e.g.). Also the predictions are based on photon statistics and do not account for uncertainties introduced in the data processing (e.g. flat-fielding errors). At the red end of the optical, especially at z, where the sky is brighter that at r and the CCD quantum efficiency is dropping, the predicted SNRs will be worse.

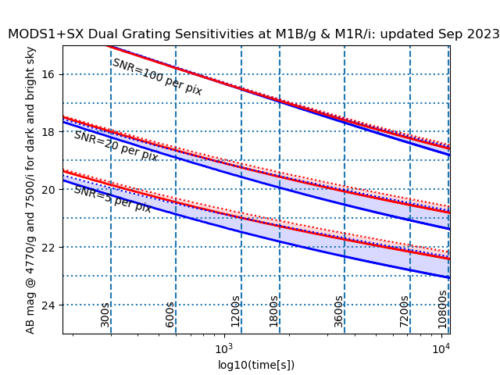
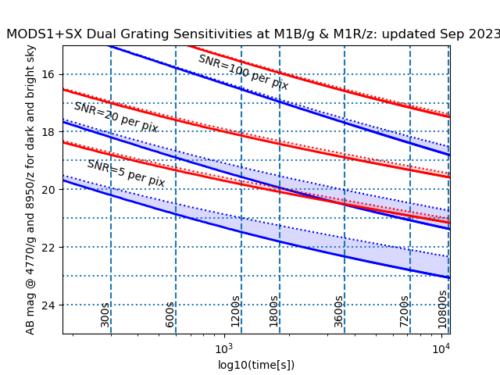
Figure 3.2 The same as in Figure 3.1, but the red channel predictions are made at i (7500 angstroms) instead of at r. At i, the sky brightnesses have been assumed to be 20.7 mag/sq.arcsec (dark) and 20.2 mag/sq.arcsec (bright).

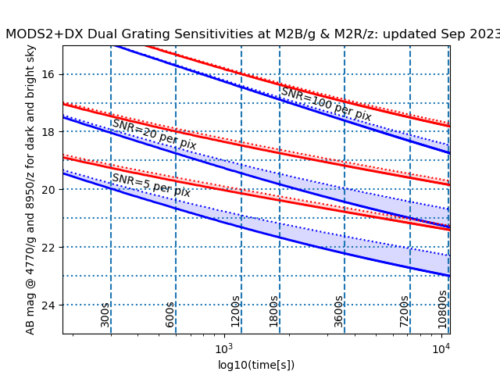Figure 3.3 The same as in Figure 3.1, but the red channel predictions are made at z (8950 angstroms) instead of at r. At z, the sky brightnesses have been assumed to be 19.0 mag/sq.arcsec (dark) and 18.7 mag/sq.arcsec (bright).

Prism Mode (not updated; from MODS1 commissioning)

The efficiency curves, exclusive of the atmosphere, for dual and direct prism modes are shown below. (Note: These are for the MODS1 commissioning data from 2011, and as for the grating and imaging modes, we can expect a decline in the sensitivity of MODS1+SX. These should NOT be compared with the efficiency curves for the grating mode in Figure 2. These curves will be updated.)

Figure 4: MODS1+LBT SX airmass=0 efficiencies for direct and dual prism modes (from the 2011 MODS1 Commissioning data).

Spectroscopic zero points as a function of wavelength for the MODS1 prism modes are tabulated below:

Table 2: MODS1 Prism Mode Spectroscopic Zero Points (based on MODS1 commissioning data 2011)

Red Prism				Blue Prism
	log Sλ ,0		Pixel		log Sλ ,0		Pixel
λ (Å)	Direct	Dichroic	δ λ (Å)	λ (Å)	Direct	Dichroic	δ λ (Å)
5000	15.722	…	2.6	3600	15.663	15.640	3.4
5500	16.178	14.978	3.4	3850	15.886	15.858	4.4
6000	16.268	16.265	4.6	4030	15.978	15.927	5.1
6450	16.301	16.293	5.9	4500	16.121	16.096	7.1
7000	16.357	16.348	7.6	5000	16.162	16.138	9.8
7450	16.365	16.368	9.1	5500	16.172	16.112	12.7
8000	16.342	16.350	11.2	6000	16.143	15.478	15.8
8500	16.307	16.297	13.3	6450	16.082	…	18.5
8800	16.264	16.252	14.6	7000	15.964	…	21.8
9750	15.740	15.692	18.9
10000	15.396	15.333	20.1

The same formula for estimating counts per exposure time used for the grating mode apply to data in this table for the prism, but bear in mind that the size of spectral pixel is a strongly varying function of wavelength. Nominal pixel sizes for each channel, δλ (Å), are given in Table 2 above.

Figure 5: For signal-to-noise ratios, SNR= 5, 20 and 100 per pixel in a prism spectrum that has been extracted over the number of rows corresponding to the seeing FWHM, tracks of AB magnitude vs time are plotted for both blue (at g_sdss/4770 angstroms) and red (r_sdss/6300 angstroms) channels.  These tracks use the MODS1 dual prism zeropoints tabulated here and have been calculated for a 1″ slit, seeing FWHM = 1″, airmass = 1 and dark (new moon, solid lines) and bright (10-d moon, dotted lines) skies. With the prisms, the number of angstroms per pixel increases with wavelength, from ~3 angstroms/pix to ~20 angstroms/pix (see Table 2 above), so while the zeropoints for the prism and grating are similar, the predicted SNR per pixel is greater for the prism than for the grating, all other input parameters being equal. The blue and red shaded regions indicate the range of magnitudes predicted for sky brightnesses from new to 10-d moon. The slit loss calculation assumes a Gaussian PSF, so for a slit width equal to the seeing FWHM, 24 percent of the light is lost. For the sky contribution, the area is the number of pixels covered by the slit width times the FWHM. The dark(bright) sky brightnesses at g_sdss and r_sdss were assumed to be 22.3(20.7) and 21.3(20.6), respectively. These are interpolated from the KPNO sky brightnesses and agree well with published dark sky values for Mt. Graham (Taylor+2004,PASP,116,762 and Pedani 2009, PASP,121,778).
To do a “bye-eye interpolation” from this plot, recall that the SNR scales with the square root of the flux and of the exposure time, although for fainter objects whose flux is comparable to the sky brightness, SNR grows more slowly. Caveat: The  predicted SNRs should be considered best-case scenarios, as there are various observational factors that can degrade the actual SNR you see in your data: transparency, slit loss, sky brightness which can change with time (in the red, for example) and with telescope pointing (looking at a low elevation target in the direction of a city, e.g.). Also the predictions are based on photon statistics and do not account for uncertainties introduced in the data processing (e.g. flat-fielding errors). At the red end of the optical, especially at z, where the sky is brighter that at r and the CCD quantum efficiency is dropping, the predicted SNRs will be worse. 

Imaging Mode

Estimates of the imaging sensitivity of MODS are based on measurements of secondary photometric standard stars in the SDSS AB magnitude system (Clem, VandenBerg, and Stetson (2008)), and, for those fields not covered by Clem et al, measurements of stars in the SDSS DR12 catalog.  By manipulation of the photometric conversion formula:

m_f  = m_f,0 – 2.5 log S_f + 2.5 log t_exp – K_f X ;

the predicted integrated counts in ADU in a filter given its SDSS AB magnitude in the filter band is given by:

 

log S_f = log S_f,0  –  0.4m_f  +  log t_exp  – 0.4 K _f X

where:

log S_f = log counts in ADU for filter f
log S_f,0 = ADU zero point (counts for m_f=0.0^mag in 1 second) listed in the table below. [log S_f,0 = 0.4 m_f,0]
m_f = SDSS magnitude in filter f in AB units
m_f,0 = photometric zero point magnitude in filter f in AB units listed in the table below.
t_exp = exposure time in seconds
K_f = estimated extinction coefficient for filter f
X = airmass at the time of the observation

Imaging Zeropoints

Preliminary photometric zeropoints in dual and direct imaging modes, derived from observations of the field of M92 on Sep 07 2023 UT and the field of M13 on May 26 2023 UT, are given in Tables 3a & 3b, along with the extinction coefficients that were assumed. Errors on the Sep 2023 data are ~0.05 mag. Table 3c lists the zeropoints that were measured from images of 4 fields with SDSS DR12 coverage observed on Feb 10 2023 UT, which were in good agreement with results based on data taken on 2021 Oct 27 UT.

Table 3a: Preliminary MODS ugirz Zeropoints from  6 Sep 2023 (after recoated SX ASM installed)

Filter	MODS1	MODS1	MODS2	MODS2	Kf (mag/airmass)
	mf,0 (mag) direct	mf,0 (mag) dual	mf,0 (mag) direct	mf,0 (mag) dual
SDSS u	25.58	25.49	25.71	25.6	0.47
SDSS g	27.29	27.23	27.29	27.21	0.17
SDSS r	26.73	26.65	27.55	27.44	0.10
SDSS i	26.50	26.52	27.33	27.33	0.05
SDSS z	25.78	25.77	26.78	26.73	0.03

Table 3b: Preliminary MODS ugirz Zeropoints from 26 May 2023 (after M2 swap)

Filter	MODS1	MODS1	MODS2	MODS2	Kf (mag/airmass)
	mf,0 (mag) direct	mf,0 (mag) dual	mf,0 (mag) direct	mf,0 (mag) dual
SDSS u	25.56	25.46	26.05	25.92	0.47
SDSS g	27.25	27.2	27.40	27.32	0.17
SDSS r	26.65	26.63	27.77	27.63	0.10
SDSS i	26.50	26.57	27.55	27.53	0.05
SDSS z	25.84	25.86	27.03	26.97	0.03

Table 3c: Zeropoints^(a) from 10 Feb 2023 UT

Filter	MODS1	MODS1	MODS2	MODS2	Kf (mag/airmass)
	mf,0 (mag) direct	mf,0 (mag) dual	mf,0 (mag) direct	mf,0 (mag) dual
SDSS u	24.80±0.13 (0.37)	24.71±0.15 (0.26)	26.11±0.05 (0.11)	26.04±0.06  (0.08)	0.47
SDSS g	26.81±0.06 (0.02)	26.75±0.06 (0.03)	27.46±0.06 (0.02)	27.39±0.04 (0.04)	0.17
SDSS r	26.41±0.09 (0.06)	26.35±0.15 (0.06)	27.82±0.03 (0.05)	27.69±0.06 (0.06)	0.10
SDSS i	26.29±0.10 (0.03)	26.36±0.15 (0.08)	27.63±0.03 (0.02)	27.60±0.04 (0.07)	0.05
SDSS z	25.67±0.10 (0.07)	25.67±0.10 (0.08)	27.10±0.05 (0.06)	27.04±0.04 (0.15)	0.03

(a) The zeropoint and error was taken to be the median of the 4 or 5 images per configuration, and the number in parenthesis represents the spread. The spread is, in most cases, smaller than the standard deviation for an image, but when it is large, as at u, it cannot be explained by residual extinction and is probably is real scatter in the very small set of stars that were detected in some fields.

The table below is based on MODS1 commissioning data taken in 2011, and these are the zeropoints used by the Imaging ETC. (We are working on a new ETC for spectroscopic and imaging modes that will use the latest zeropoints – 2023 June 05). When planning imaging, for science or for spectroscopic acquisition, a good rule of thumb is: The peak pixel will saturate on an r = 15^th mag in 30 sec in 0.6 arcsec seeing.

Filter	mf,0 (mag)	Kf	log Sf,0
SDSS u	25.68±0.12	0.47	10.25
SDSS g	27.38±0.03	0.17	10.94
SDSS r	27.24±0.03	0.10	10.90
SDSS i	27.21±0.04	0.05	10.91
SDSSz	26.41±0.04	0.03	10.58

 

 

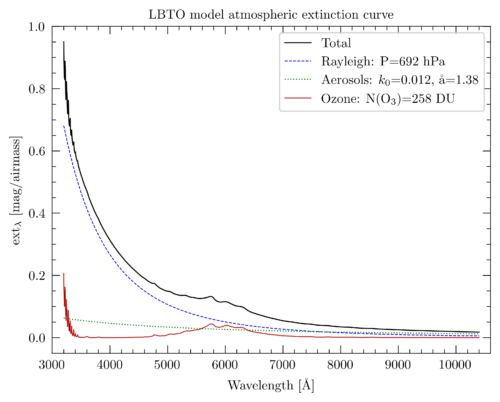
Revised Model LBT Atmospheric Extinction

Lacking an empirical extinction curve for the LBT site on Mt. Graham, a model extinction curve was constructed in 2012, based on the KPNO and Paranal extinction curves scaled to an elevation of 3200-m, assuming an atmospheric scale-height of 7000-m.

A revised model extinction curve for the Mt. Graham site (Pogge et al, in prep) is now available at this link. This revised curve is sampled every 0.5-angstrom and the total extinction (2nd column) is broken down into the separate Rayleigh, Aerosol and Ozone contributions in columns 3, 4 and 5, respectively. In comparison with the 2012 one, this updated one resolves the ozone Huggins bands (λ < 3500Å) and Chappuis bands (λ ~ 4500-8000Å) and fills in the spectral region from the red optical to the near-IR. The general shape is in good agreement with the old one.

The plots below compare the revised model extinction curve with the 2012 one (top) and the contributions of the Rayleigh, Aerosol and Ozone components (below). The Rayleigh component assumes a mean atmospheric pressure, 692 hPa (+/- 6 hPa), derived from LBT weather station measurements over 2023 and the first 8 months of 2024. The aerosol contribution assumes the Angstrom 1964 parametrization with aerosol optical depth at 1-micron of k0 = 0.012 and Angstrom exponent a=1.38. And the ozone is the Reference Forward Model with reference column density of 258 DU (Dobson Units).